The HyperSphere, from an Artistic point of View part of Rebecca Frankel's Web Site

How do we view objects in four dimensions?

The difficulty with viewing an object on a hypersphere is not being able to see it -- it is, after all, a three dimensional object living in a three dimensional space -- but being able to see all of it. It is an object that lives in a curved space, and we want to see it flattened out. Imagine, by analogy, a flatlander viewing something drawn on the surface of a two-dimensional sphere. He could walk around on the sphere's surface, looking at the object, but he could never see all of the sphere that way, because there would always be part of it beyond the horizon. So any object that was drawn on the whole sphere would only partially be available to view. Alternately, as Edward Abott famously describes in his book Flatland, he could view the sphere as it passes through his flat space. However, it takes quite an act of imagination to reconstruct a full sphere from its successive cross-sections. This isn't impossible: if we are willing to accept some distortion we can project the whole surface of the sphere onto three dimensional space.

So what are we looking at?

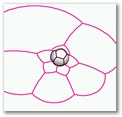
Suppose a flatlander wished to view structures on a sphere from his comfortable planar home. He could arrange for a transparent sphere to sit on the plane with a light placed at the top, so that all the circles drawn on the sphere would cast shadows down onto his plane. Now these shadows would be distorted images of the original circles, so the images would still seem unnaturally large in the distance, but angles and approximate shapes would be preserved. The flatlander could sit well away from the point where the sphere rested, and watch the play of shadows as the sphere rotated.

It is easiest to understand this process by looking at the analog in two dimensions. Above we see a sphere with the edges of dodecahedron projected onto it (it is as if we took a dodecahedron with stretchy faces and blew it up unto a sphere, like a soccer ball). Now imagine the sphere is transparent and we put a light on the top. We can shine the light down on the plane and see the shadows of the edges of the dodecahedron on the plane. The name for this method is stereographic projection.

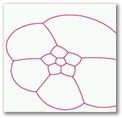
Above right we see the twelve faces of the dodecahedron projected on the plane this way. Can you pick them out? The projection of the bottom face is hidden underneath the small sphere; the second row of five faces projects to the small five-pointed flower image; the next row of five to the bigger more curved images, and the final top face is projected out to infinity, forming the "background" for the figure. Since this might be difficult to see with the sphere in the way, I have provided another image of the projection, with the original sphere removed, to the left.

This projection distorts our shape, the dodecahedron, but in a predictable way. All the faces of our dodecahedron are identical regular pentagons, but in the projection they look quite different from each other. In particular, the images towards the center look abnormally small, while the images towards the edges seem abnormally large -- and any face that passes through the projection point gets blown up infinitely large and becomes the "background." Also, straight lines are no longer straight: they become sections of circles, and as they approach the edges of the projection they become more and more noticeably curved. However, angles are preserved, so it is not too difficult to see that we are looking at pentagons -- distorted, for sure, but still most definitely pentagons.

The advantage of this projection is that it would help a flatlander to get a concept of a three-dimensional dodecahedron without having to mentally reconstruct slices of the body as it passed through his space. He could wander among the shadow lines on his plane, and if he had the imagination to mentally correct for the funny distortion of lines and areas, he could get a sense of a figure with twelve equal pentagonal faces wrapped on a sphere. Maybe he wouldn't know everything there is to know about a dodecahedron, but he could learn many of the most important things: how it was connected, its regularity and its symmetry. He can still see the essential three-fold and five-fold symmetry of the dodecahedron. We can imagine a flatlander looking at this projection and intuiting from it an idea of the original object. In particular, this projection makes it possible to intuit the inherent symmetry of this object. These properties are much harder to convey using the two-dimensional slices model.

Our next step is to assign the task we have just watched our hypothetical flatlander perform to ourselves. We want to wander among the shadows of the "faces" of a four dimensional polyhedron and reconstruct in our imagination a concept of its connectedness, regularity and symmetry. But in four dimensions an "face" will actually be a body, a three-dimensional object. Look up above at the green object pictured at the start of this essay. Does it have a form that looks familiar? See the small inner star, and the larger more curved outer leaves? Can you see the underlying regularity, the consistent pentagonal shapes, with, now another layer of consistent dodecahedrality? It looks like our original projection, except that now it is more complex.

The image above is, as you might have guessed, the projection of the faces of a four-dimensional analog of a dodecahedron. We are looking at the "shadows" of the faces of this figure, which are ordinary three-dimensional dodecahedron. We aren't seeing all of the faces: if we showed them all we wouldn't see anything but the inside of the one which projected to infinity. Some faces have been removed to make the picture easier to view -- in fact, we are looking at exactly half the faces. To imagine the whole shape one would have to imagine another exact copy of this figure fitted into this one -- but don't worry too much about trying to bend your mind around this concept here, for we will talk more about it later. The only thing that matters now is to get a sense of the regularity underneath the distortion.

Above we see another image of a selection of faces from the 4D dodecahdral polytope which might be easier to interpret. The solid "faces" of this hyper-dodecahedron have been pulled away from each other slightly, which makes it easier to see that they are all reasonably similar dodecahedron. The big mesh sphere is the projection of the "equator" of our sphere. The middle dodecahedron on the section to the right of the "equator" lies at the north pole, while the middle one at the left lies on the south pole. Perhaps this view makes the underlying regularity clearer: it is easier to see that the two halves are basically equal, with only slight distortion because of the projection.

Let us look at another example, to get a sense of how this works. Above we see a pair of two-dimensional projections, this time of an icosahedron. Notice the triangles have changed, in the projection, into another characteristic petal shape. They distort slightly as the sphere is rotated under the light that sits at its top. Notice the characteristic shapes of the distorted triangles. Now, we can see a similar characteristic shape in the image above, which is a projection of a four-dimensional polytope called the 24-cell. In this image, only alternate solid "faces" are pictured, so we can get an idea of the shape. Note the faces of this shape look like three dimensional analogues of our original petal-shaped triangles. By comparing the projections in these different dimensions, we can gain an intuition which allows us to imagine the form of the original four-dimensional polytope.

How the four dimensional sphere is different.

In particular, to give you an idea of the new dimensions in hairstyle available in four dimensional, note that there is more than one way to divide all the "hair" into two sections, i.e. to make a part. You can divide it much the same way one would make a part on a three-dimensional head. In this case the dividing line of the "part" would not be a circle drawn on the "head" -- it would be a sphere. Since we are dimension higher, the "part" is also an object of one higher dimension. To get an idea of how this looks (in projection) we show above an image of a four sphere parted -- into six equal sections, not two. This is how one would make parts if one was preparing to braid six cornrows on the head of our four-dimensional woman. Notice how the dividing surfaces are portions of spheres.

This is not the only way to divide the sphere, however. We can also divide the hair into two sections using a torus as our dividing line. That is to say, we can also find a torus that cuts the four dimensional sphere into two pieces. Above you can see images of the dividing torus (projected). The dividing torus has been cut into ribbons, so we can see it projected in our space. The ribbons on the torus suggest how to comb the hair on a sphere: we can make the dividing line of the "part" into a torus -- and it is possible to comb the hair on a torus. I have included a picture of the flow along this torus, from two different perspectives. Because it would be hard to see individual "hairs" in this flow, I have taken two equally spaced "hairs" -- or "fibres" as they are called in mathematics -- and connected them with a surface to make a ribbon, as I mentioned before. So we see ribbons twisting around a projected torus.

Look carefully at these ribbons. Notice that every single one has a twist in it. If this is hard to see in these small images, click on the picture to magnify it. The flow that wraps smoothly around the torus is made up of circles that do not intersect, and each circle is linked inside every other circle exactly once. So when we connect two circles as a ribbon, these ribbons have an elegant twist to them. These linked circles and single twists are ubiquitous in images of the four dimensional sphere. They add particular harmony to the images. Below are examples drawn with more distance between the connected circles, so the ribbons are drawn wider. In these images, the twist is more visible.

Now that we have looked at the torus that divides the sphere in half, and the family of circles in which each circle links every circle exactly once, I want to return again to the subject of four dimensional polyhedra, which are often called "polychora". The surprising property of the four dimensional sphere -- that you can "comb its hair" -- applies also to the polyhedra in four dimensional space. That is, just as you can find a smooth flow that takes the whole sphere to itself, you can also find a flow of the discrete faces of a polychora onto each other that brings it back to itself. That means it is interesting to look at subsets of the faces which make up elements of the flow. This is an entirely emergent property of four dimensions -- by contrast, there aren't any subsets of the faces of a three dimensional polyhedron that have any particular physical or mathematical meaning. Four dimensions is different, however. This essay can not explain the full story of the exceptional properties of the four dimensional sphere, but it aspires to give you a taste for them.

Polyhedra, revisited.

For example, let us return, as promised, to consider the half hyper-dodecahedron which decorates the top of this article. There are one hundred and twenty solid faces in this four dimensional version of the dodecahedron, and I show a solid made up of half of them, sixty in all. How did I choose this particular half-object? Well, considering the earlier discussion, you may guess that I found a way to part the dodecahedron into two solid tori, in much the same way the sphere itself can be cut in half with a torus. Now the next natural question is: can we find a smooth flow of linked circles that wraps around this torus? Yes we can: at left we see two linked circles which have been selected from sixty faces of our half-dodecahedron. Each circle is made up of ten solid faces. The whole tori that makes up our half-dodecahedron contains six of these linked circles. If you look carefully again at the image of the whole tori, perhaps you can pick out the circles that wrap around the figure. These characteristic linked circles show up everywhere in images of the denizens of four dimensional space, and lend the images their particular harmony.

The hyper-dodecahedron is not the only four dimensional polytope to contain these characteristic linked rings. Below we see an image of the characteristic linked rings from another four dimensional figure. This polygon is harder to name, because it does not have a three-dimensional analogue. Its faces are made of truncated cubes, and it has forty-eight cells in all. Just as was the case for the hyper-dodecahedron, we can pick out a torus made up of half of the faces. We see those twenty-four faces pictured below. In order to see the faces more clearly, we have drawn them slightly smaller, so they pull away from each other. This makes it possible to actually see all twenty-four faces. As you can see, this solid tori are made up of three linked rings of eight cells each. In this case, it is actually possible to see how the entire torus is divided into these linked rings.

The linked rings show up in another manner as well. Remember the 24-cell that we mentioned earlier? We discussed it when we were explaining how to understand the projection into three dimensional space. This is a polygon made up of twenty-four tetrahedral faces. The image we showed of it earlier was the snaggle-toothed view: we drew every other point. Now we show an image of one single ring of six faces chosen from the twenty-four. The equator of the sphere is also drawn as a mesh in this image, to help us orient ourselves. Now look carefully at this image. Since the twenty-four cell is a relatively small polygon, these faces are relatively large, and the whole ring looks like a solid torus on its own. Notice that the edges of the tetrahedra describe arcs which continue from one cell to the next. These arcs make up circles that wrap all the way around our small torus. So again we see the characteristic circles that wrap around a torus, this time in yet another context. These are circles drawn on a single ring of faces, which is itself a circle that entwines with other circles. Objects embedded in the four dimensional sphere contain circles within circles within circles. This property gives them a particular harmony and beauty.

What else can we view?