 |

|

|

|
 |

|

|

|
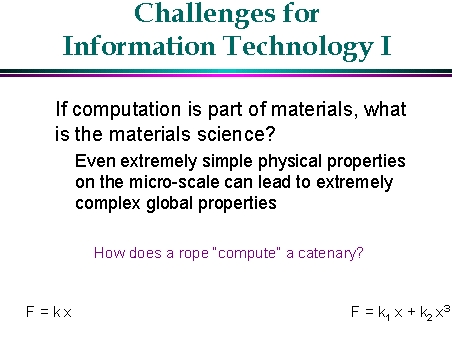
For example, consider even a very simple mechanical system such as a rope. Ropes are very simply described at the micro-scale as a linear spring,
F = k x.
We know from differential equations how to map from that local description to the overall shape of the catenary that a rope takes on under the influence of gravity. MEMS lets us modify the local properties of a system. The tricky part is that if we make even a very slight change to the local behavior, such as changing from being a linear spring to a stiffening spring (essentially adding a cubic term), then it's called Duffing's equation, and there are people who spend their whole lives studying it, trying to understand how to map from this simple local property to the global shape.